Impulse: The King of Performance Metrics

The world of player development is convoluted, with a litany of different systems and viewpoints biased from everything from biomechanics, to workload management, to neural pathways and dozens of other factors. All of these viewpoints can provide valuable secondary context to performance, but the majority of sporting activity really comes down to these two things, applied in a variety of ways:
-
You need to change the displacement of either your center of mass, or that of a separate implement, to get it to a more desired location on the playing field.
-
You typically need to be able to do this at very high velocities, and be able to manipulate the direction and rate of change of those velocities to avoid opponents, or get in the way of their inversely oriented displacement objectives.
First, let’s start with a brief overview on Newtonian Mechanics. For our purposes (not diving into relativity or quantum mechanics here) we can split this branch of physics into Kinetics and Kinematics, two main concepts that allow us to understand sports performance.
Kinetics
Kinematics is the branch of mechanics that describes the motion of objects without considering the kinetics that caused the motion. It focuses on quantities like displacement, velocity, and acceleration, which are also all vector quantities (have both magnitude and direction).
Kinematic Quantities
Displacement: The shortest distance from an object’s initial to final position, with respect to direction.
Velocity: The rate of change of displacement with respect to time.
Acceleration: The rate of change of velocity with respect to time.
When it comes to making connections between displacement, velocity and acceleration, it can also be very useful to understand the calculus principles of Integrals and Derivatives.
A derivative measures the rate of change of a quantity, giving the slope of a function at any point. A integral does the opposite, calculating the accumulation of a quantity by measuring the area under the curve.


Here are paired kinematics graphs of simple motion examples you may see in real life. Take a moment to apply the calculus principles listed above to make connections between displacement, velocity, and acceleration for each example. This derivative relationship between velocity and acceleration is the one people seem to have the biggest issue understanding. To make things simple, if the directions of velocity and acceleration are the same, regardless of magnitude, the object is speeding up. If they are opposite, the object is slowing down.
Impulse-Momentum Theory
Let’s start here by defining Impulse and Momentum.
Impulse (J) is defined as the product of the net force acting on an object and the duration of time that force is applied. So in short, it is the total area underneath the Force-Time curve (an integral, just like we talked about earlier). J=FΔt.
Momentum is defined as the product of an object’s mass and its velocity. .It represents how much “motion” an object carries. The main goal of a thrower, for example, is to change the implements momentum as much as possible. p=mv.
Impulse–Momentum Theorem states that the net impulse on an object is equal to its change in momentum: J=Δp=mv(final)−mv(initial). In the majority of non-contact sporting contexts, mass is constant, so we can write the formula like this-
FΔt= m(vf-vi).
This is a perfect example of forward dynamics at work, going from force (kinetics) to velocity (kinematics). So to be able to change the momentum of our center of mass, or the center of mass of a separate implement, the goal is to create as much positive impulse as possible.
Side Note- The fact that Impulse is a integral is exactly why it is so reliable, because it is a full look at the area under the force-time curve. This is why more derivative-based metrics like peak power are much more variable and not as reliable, because they only exist at one specific point on the force time curve. A longer write up on this by Dr. Jason Lake can be found here.
Impulse Maximization Strategies
In order to create as much area under the force time curve to change their momentum, there are three main strategies athletes can employ. These strategies do not exist on a binary, all three strategies need to be be used to maximize impulse.
1. Create greater peak forces, allowing for a taller force-time curve. This can be done by improving neuromuscular output, and improving coordination to take advantage of more favorable leverage positions.
2. Improve the rate of force development to which they reach peak force, allowing for steeper sides of the force-time curve. Likewise, this can be done by improving neuromuscular output, and improving coordination to take advantage of more favorable leverage positions.
3. Increase the width of the force-time curve by lengthening the duration of the impulse. This strategy has is more limited compared to the other two, as the majority of sporting movements are placed under severe time constraints. A great example of a positive way to employ this strategy would be imprioving a throwers horizontal abduction and external rotation of the throwing arm will lengthen the amount of time they have to put force into the implement, increasing the impulse.
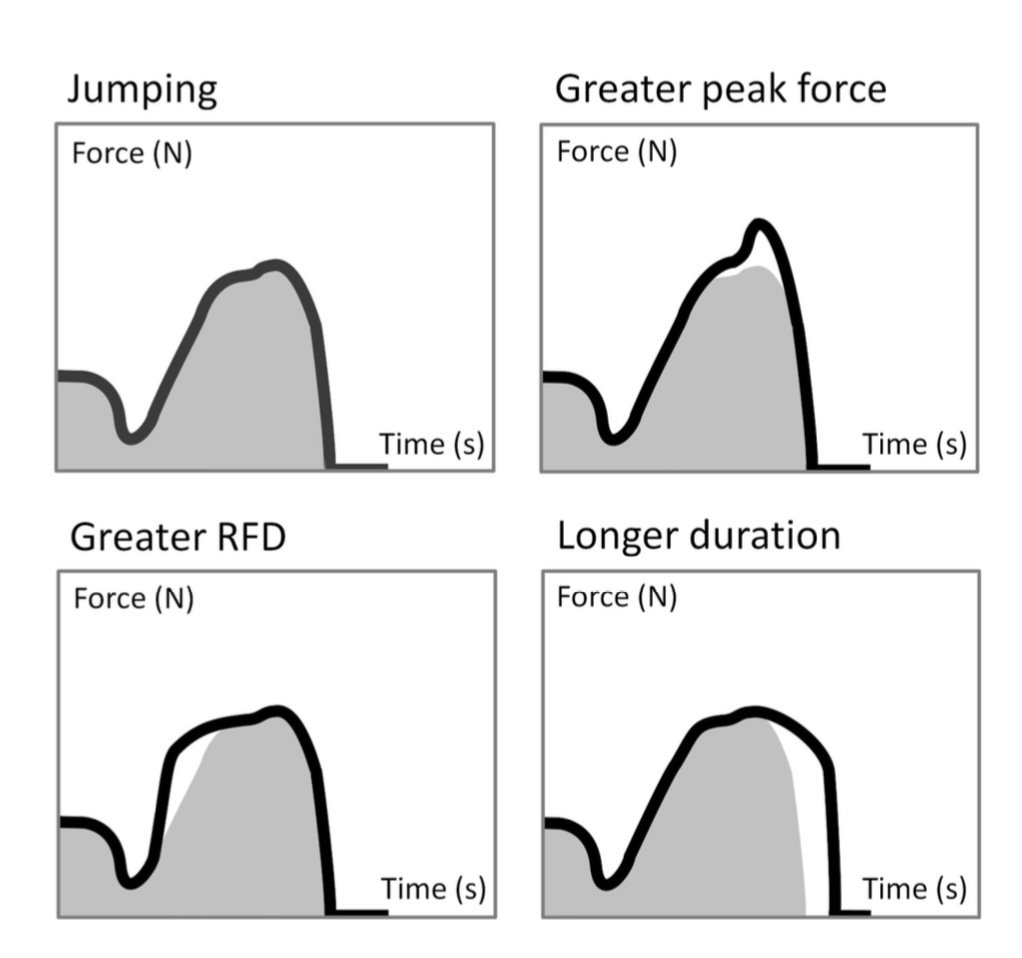
Cleather, D. (2021). Force: The biomechanics of training.
An example of a Drop Jump (Top) and Depth Jump (Bottom). The Drop Jump biases higher rate of force development, with significantly lower ground contact times, resulting in a much sharper force curve. The Depth Jump has longer ground contact times, which allows for more total impulse under the curve, creating a higher jump. Neither is necessarily “better”, they’re just two different strategies for producing impulse.
So when trying to improve skill outputs, its a question of improve the athletes ability to express impulse within the constraints of the skill. The question then is “how do we improve the athletes lowest hanging fruit when it comes to impulse strategies, while maintaining and improving on the strategies they currently do well?”.
One of the four main buckets of constraints you can place on the throw is changing the mass of the implement.
One of the foundational principles of variably loaded ballistic work is that it provides varied movement strategies due to the difference in the implements inertia⬇️ pic.twitter.com/B02Y88XOym
— Brice Crider (@Crider_HP) January 11, 2025
From there, we can start to look at some of the underlying reasons (anatomy, physiology, etc.) why the athlete produces impulse the way they do, and begin to work on some of those pieces to improve the athletes outputs. This is where most people have it backwards. They start with more subjective looks at A&P, and try to use the information to improve sports performance, without really ever quantifying outputs, both in general and in specific.
Clayton Thompson wrote a great blog on this exact subject- The Case Against ISA.
Creating Impulse in Sport
Muscle Driven Outputs – Push and Pull
The push strategy is mainly driving by the contractile tissue (muscle) changing length, allowing the athlete to manipulate their center of mass using ground force reactions. Especially in something like the jump where the load is centered within the athletes center of mass, the athlete will employ a combination of hip and knee flexion/extension to accomplish the task of putting force into the ground.
Because these forces are mainly created by contractile tissue, there is a delay caused by the need for signaling at the neuromuscular junction, actin-myosin binding, and finally contraction, which biases a wider impulse curve. This isn’t to say that collision based movements don’t involve muscular contraction, they’re just less biased to being reliant on muscular output.
A countermovement jump is a good look at how muscular forces are used to create the positive impulse that changes an athletes momentum in the vertical direction.
Collision Driven Outputs (Bounce and Block).
A collision is an event in which two or more objects come into contact and exert forces on each other over a short period of time. Many high speed sporting outputs are reliant on managing these collisions using both active and passive structures to be able to efficiently transfer energy up the kinetic chain. These collisions can be managed in two main ways, by bouncing or blocking. The main way to differentiate between is by looking at the stiffness of the collision, also known as the collision coefficient.
The collision coefficient gives a look at the conservation of momentum. In a perfectly elastic collision, both momentum and kinetic energy is conserved, creating an opposite “bounce”. In a perfectly inelastic collision, Momentum is conserved, but kinetic energy is not, causing a “stick” or “block”. Because the elastic collision is created by deformation of one or both of the physical bodies involved in the collision, the rate of force development is lower than in the inelastic collision.
An example of bounce and block strategies shown by dropping a 2.5 lb plate and a rubber ball-
The bounce strategy is far more elastic, allowing for some deformation of the limbs to smoothly transition from yielding and braking into propulsion.
The block strategy is far more inelastic, aiming to bring a sudden halt, which is very useful for rapidly stopping certain pieces to send energy up the kinetic chain.
All of these biomechanical impulse strategies don’t exist on a binary, but rather exist on a spectrum. The majority of sporting actions require the athlete to use all four strategies simultaneously as they express force and send energy from piece to piece through the kinetic chain.
Sprinting employs push, pull, and bounce strategies to produce high peak forces in a small time window.
Conclusion
If there’s one takeaway from this entire piece, it’s that analysis of sports performance should start by looking at what actually moves the needle: force production.
Understanding the newtonian mechanics behind impulse will give you a more foundational and holistic backing to your training interventions, so you can spend less time guessing, and improve performance.
Happy Hunting.
Sources-Cleather, D. (2021). Force: The biomechanics of training.
Comment section