Using PULSE to define throwing workload
DISCLAIMER: At the time of usage and data collection, PULSE was known as Motus. We will be referring to it as PULSE throughout this blog.
The foundational concept behind the use of the PULSE sensor is “workload”—but what exactly does workload refer to?
In a general sense, workload describes the stress from each throw an athlete makes. So, does that mean that workload is just a measure of the number of throws an athlete makes in a given game? Not quite.
What typical in-game pitch counts fail to account for is the varying workloads between game days, as well as the variance in pregame workloads between athletes. For example, let’s evaluate two hypothetical athletes:
Player A
Long tosses out to 300 feet
Throws a 30 pitch pregame bullpen
Throws 85 pitches in the game
Player B
Long tosses out to 150 feet
Throws a 20 pitch pregame bullpen
Throws 85 pitches in the game
Using pitch counts, these athletes would be considered to have the same workload; however, we can see that they make a drastically different number of throws.
Okay, you may be thinking, so we should just track the total number of throws athletes make each day? Not quite.
Counting the total number of throws each day would certainly be better than just pitch counts in-game. Unfortunately, this method would still fail to account for the varying intensities between various throws. Is a throw at 100% intensity on the mound worth the same as a 50% intensity throw at the beginning of catch play?
With the use of the PULSEthrow sensor, we are able to measure the amount of valgus torque placed on the elbow with each throw. These values have also been validated against data generated at our in-house biomechanics lab at Driveline.
A throw with a stress value of 60 Nm will have a much different impact on a 16-year-old high school kid who is 6-foot and weighs 150 pounds, than on a 28-year-old professional pitcher who is 6-foot, 200 pounds. To take this into account, each throw is normalized for the given athlete’s height (in meters) and weight (in kilograms).
Various loads have different impacts on our bodies over time. A squat of 400 pounds is going to leave me sorer than if I just squatted 200 pounds twice. In throwing, this would be equivalent to me throwing a ball with a peak elbow valgus torque of 100 Nm in comparison to two throws with a peak valgus torque of 50 Nm.
The One Throw Workload Formula
Research by NASA into the effect of exercise on bone loading and its impact on “Daily Load Stimulus” has shown us that the stimulus from exercise is not linear with the load placed upon the body. However, this research provided us with an exponential weight to apply to the load of each throw. Below is the resulting One Throw Workload formula.
The above formula tells us that to find the workload units for an individual throw we:
- Measure the peak amount of valgus torque applied to the elbow during the throw in Newton-meters (Nm).
- Divide this value by the given athlete’s height (in meters) times weight (in kilograms).
- Take this newly calculated value to the power of 1.3.
We are left with the workload units for each individual throw. Now let’s compare the workloads of two different throws again. We will assume this athlete is 6 feet tall (1.8288 meters) and weighs 200 pounds (90.7185 meters).
Whereas before the 100 Nm throw was considered to have simply two times the workload as the 50 Nm throw, the 100 Nm throw now equates to roughly 2.45 throws at 50 Nm.
Within the PULSEthrow app and PULSEdash, certain throws are tagged as “high effort” throws. This designation is not meant to be good or bad but is simply used to provide further insight into your throwing habits each day. To begin, the elbow valgus torque from all throws in the past two weeks that were not simulated, weighted balls, or outliers are summed. In other words, the number of throws with a 5-ounce baseball were measured in real-time over the past two weeks.
IF there are greater than 50 throws remaining:
- The 5 highest torque values are chosen.
- The average of these 5 throws is found.
- The threshold for “high effort” is determined as 70% of the average.
IF there are less than 50 throws remaining:
- A default value is assigned based upon:
- Athlete height
- Athlete weight
- Athlete level of competition
The One Day Workload Formula
As we touched on previously, each throw in a given day is not performed at the same intensity. An athlete’s first throw of the day playing catch is likely not going to be as stressful as their first pitch in the game. To find the workload units within each day, we find the sum total of all one throw workloads within that given day. We title this metric One Day Workload.
The above formula tells us that to find the workload units for a given day we:
- Calculate all one throw workloads within that day.
- We then find the sum of all given throws by adding together the workload from the first throw of the day (throw=1) until the last throw of the day (throw=n).
The resulting one day workload gives us better insight into how each day’s throwing is impacting the athlete. While making more throws in a given day will accumulate workload within that day, throw count is not necessarily indicative of workload. Different throw counts can result in the same one day workload and the same one day workload can be reached with a number of different throw counts.
Why is one day workload of importance? By calculating the workload units from a given day, we can also evaluate trends in an athlete’s workload over time—both recently and over longer periods of time. With PULSEthrow, we are able to find each athlete’s Acute Workload and Chronic Workload. Acute workload refers to the average one day workload for a given athlete over the previous nine days, whereas chronic workload refers to the average one day workload for the last 28 days.
The condition of your arm is not simply impacted by the workload from that given day but is also impacted by the previous days you’ve thrown. If we simply took the average for the previous nine days, each day within those nine days would hold the same weight. Realistically, throwing today is going to have a larger impact on your throwing tomorrow than your throwing from eight days ago. To factor for this, PULSEthrow uses a weighted moving average for each one day workload when calculating an athlete’s acute workload. This is accomplished by the use of “Acute Kernels,” in which the workload from the most recent day is multiplied by 1.3, and workloads from previous days are multiplied by lower values, such that eight days prior is multiplied by 0.7.
Weighted Average One-Day Workload
Once we have determined the weight of each one day workload and found the corresponding values, we must then sum these values. After summing these values, the total must be divided by a number of days, “N,” to find the weighted average one day workload. Typically, N would equal nine since that is the number of days we are evaluating; however, during the first two weeks of assessing acute workload, we use a “dynamic divisor” in its place to make the values more usable. The dynamic divisor, in this case, ranges from three to nine days. On the first day N=3, and on each subsequent day, N increases by one until reaching 9 on day 7 where it will remain. This results in the following equation for acute workload during the first two weeks:
The above equation tells us that to find the workload units for acute workload we:
- Calculate all one day workloads within the past nine days.
- Multiply each one day workload by its corresponding acute kernel.
- Most recent day (Day 0): One Day Workload multiplied by 1.3
- One day ago (Day -1): One Day Workload multiplied by 1.225
- Two days ago (Day -2): One Day Workload multiplied by 1.15
- Three days ago (Day -3): One Day Workload multiplied by 1.075
- Four days ago (Day -4): One Day Workload multiplied by 1.0
- Five days ago (Day -5): One Day Workload multiplied by 0.925
- Six days ago (Day -6): One Day Workload multiplied by 0.85
- Seven days ago (Day -7): One Day Workload multiplied by 0.775
- Eight days ago (Day -8): One Day Workload multiplied by 0.7
- Add all the subsequent one day workloads together.
- Divide the sum by the corresponding acute divisor.
- After 1 day of throwing: Acute Divisor = 3
- After 2 days of throwing: Acute Divisor = 4
- After 3 days of throwing: Acute Divisor = 5
- After 4 days of throwing: Acute Divisor = 6
- After 5 days of throwing: Acute Divisor = 7
- After 6 days of throwing: Acute Divisor = 8
- After 7 days of throwing: Acute Divisor = 9
We are left with the value for the weighted average workload units for the past nine days, acute workload within the first two weeks for an athlete. After the first two weeks, we are able to use a static divisor, 9. The corresponding equation is used for acute workload following the first two weeks:
The above equation mirrors the equation used during the first two weeks for Steps 1, 2, and 3. Once the sum of the weighted one day workloads is found, it is divided by 9 each day, rather than ranging from 3 to 9.
Acute workload can be used to tell us about an athlete’s throwing habits in the short term. When used in conjunction with chronic workload, these measures can help us to evaluate how similar or different these workloads are over time.
Calculating Chronic Workload
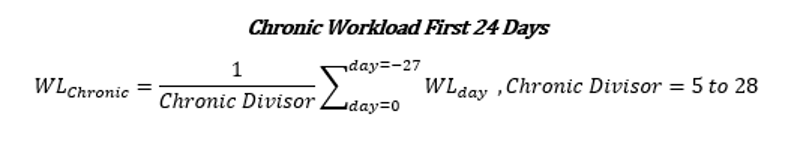
Before we can compare acute and chronic workloads, we must first calculate chronic workload. Previously we mentioned that chronic workload describes an athlete’s past 28 days of throwing. Unlike acute workload, chronic workload is not a weighted average but a rolling average of one day workloads over the past 28 days. Similar to acute workload, we initially use a dynamic divisor for chronic workload to make the values more usable. The dynamic divisor for chronic workload ranges from 5 to 28 days and is used for the first 24 days before the use of a static chronic divisor. The following equation is used for chronic workload during the first 24 days:
The above equation tells us that to find the workload units for chronic workload we:
- Calculate all one day workloads within the past 28 days.
- Most recent day (Day 0)
…
-
- 27 days ago (Day -27)
- Add all the subsequent one day workloads together.
- Divide the sum by the corresponding chronic divisor.
- Day 1: 5
- Day 2: 6
…
-
- Day 24: 28
The result is the value for chronic workload. Past the first 24 days, we are able to use a static chronic divisor, 28. The corresponding equation is used for chronic workload moving forward…
The above equation mirrors the equation used during the first two weeks for Steps 1 and 2. Once the sum of the one day workloads is found, it is divided by 28 each day, rather than ranging from 5 to 28.
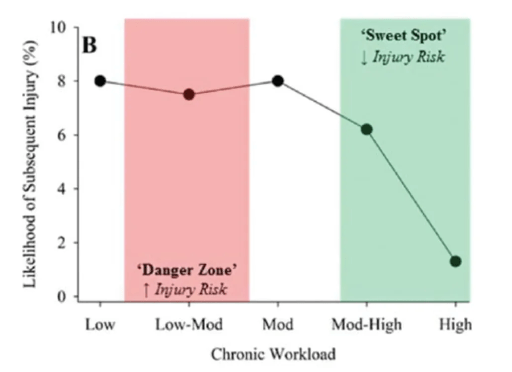
Chronic workload tells us about an athlete’s throwing habits in the long term and can be considered a measure of general throwing fitness. Again, when used in conjunction with acute workload, these measures can help us to evaluate how similar or different these workloads are over time. Higher levels of chronic workload have been associated with decreased risk of injury and also allow for greater amounts of acute and one day workload without increasing acute to chronic ratio—which we will discuss next.
Acute to Chronic Workload Ratio
The last metric we will discuss is acute to chronic workload ratio. Acute to chronic ratio tells us how similar an athlete’s workload over the previous nine days is in comparison to their workload over the previous 28 days. This ratio is found using the following equation:
An acute to chronic ratio of 1.0 would mean that the athlete’s average one day workload during those two spans was identical. An acute to chronic ratio greater than 1.0 indicates that the athlete’s current throwing workload is higher than it has been previously. Lastly, an acute to chronic ratio less than 1.0 indicates the athlete’s throwing workload is less than it has been previously.
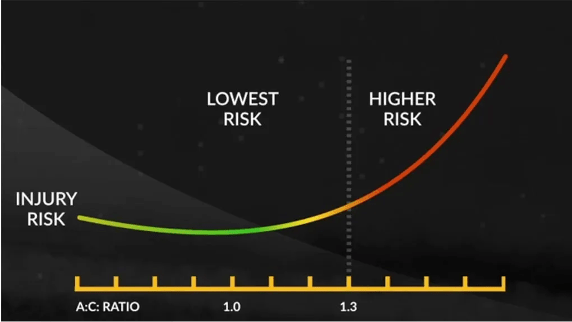
To increase an athlete’s chronic workload, the athlete must have an acute to chronic ratio greater than 1. Previous research has shown us that once acute to chronic ratios get too high athletes tend to show an increased risk of injury. The result of this research was that acute to chronic ratios in baseball athletes higher than 1.3 resulted in their being more likely to sustain an upper-body injury.
An acute to chronic ratio of 1.3 would indicate a 30% increase in the average one day workload over the most recent nine days in comparison to the previous 28 days. This is important to note, as athletes, pitchers especially, build up throwing volume and intensity in preparation for their seasons.
While we must have an acute to chronic ratio higher than 1 in order to increase an athlete’s chronic workload, we must not increase this workload too quickly or else risk significantly increasing their risk for injury. This is not to say that maintaining an acute to chronic ratio under 1.3 reduces the risk of injury completely, but it does seem to help.
On the flip side, having an acute to chronic ratio that is too low can also be detrimental. The suggested range for acute to chronic ratio is therefore 0.7 to 1.3.
By using PULSEthrow and PULSEdash, we are able to better understand the impact various throwing workloads have on baseball athletes. We can objectively measure the amount of elbow valgus torque generated during each throw and then assign the throw a value based upon the athlete’s height and weight. From this one throw workload value, we can determine one day workloads for various throw counts and intensities.
These one day workload values are then transformed into acute and chronic workloads that tell us about an athlete’s throwing workload in both the short term and long term. These acute and chronic workloads allow us to determine the acute to chronic workload ratio for the given athlete—which has been shown to signal an increase in the risk of potential injury.
Traditional thought may be that workload is measured as a means to reduce the amount a pitcher throws. In reality, if monitored and progressed appropriately, objectively measuring pitchers’ workloads could allow us to have pitchers handle larger workloads more safely.
Written by Devin Rose

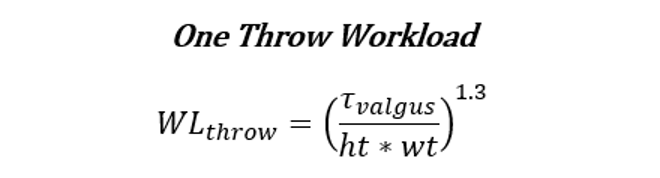


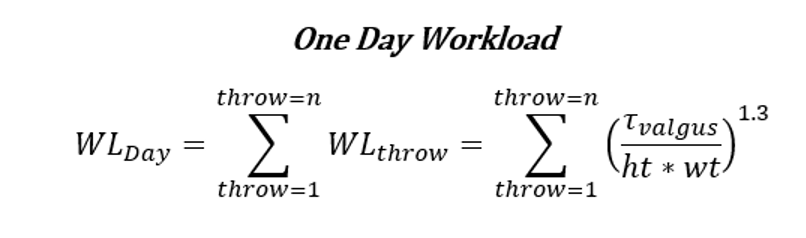
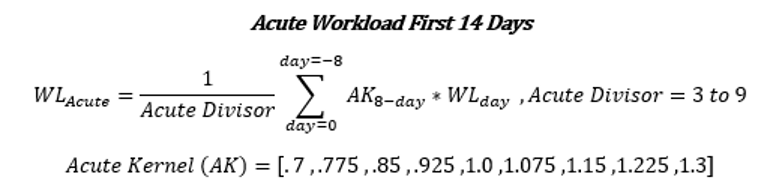
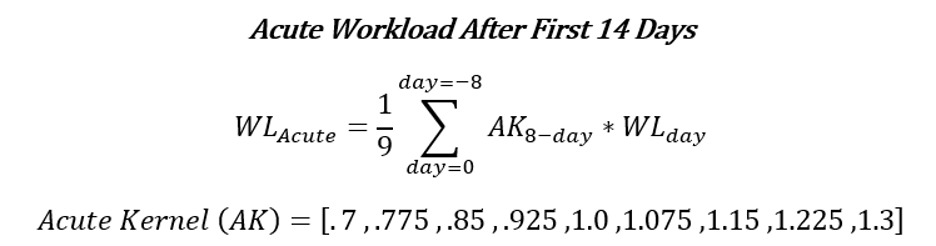
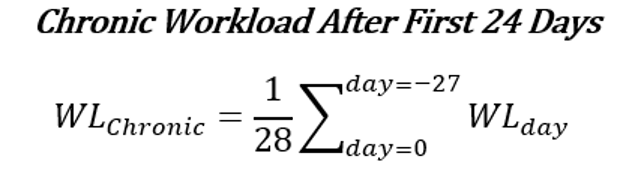
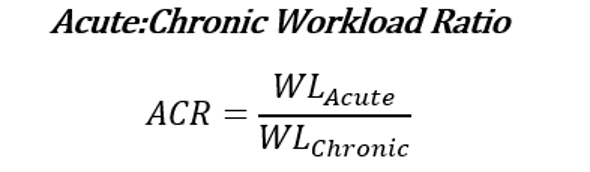
Comment section